History of Computers

Table of empoyees

What is a Computer?

A computer is an electronic machine that collects information ,stores it, processes it according to user instructions, and then saves returns the result.

A computer is a programmable electronic device that performs arithmetic and logical operations automatically using a set of instructions provided by the user.

Computers can be different:

1. Telefons
2. Tablets
3. Navigation machines

Early Computing Devices

People used sticks, stones, and bones as counting tools before computers were invented.
More computing devices were produced as technology advanced and the human intellect improved over time.
Let us look at a few of the early-age computing devices used by mankind.

1. Abacus

The abacus (plural abaci or abacuses), also called a counting frame, is a calculating tool which has been used since ancient times. It was used in the ancient Near East, Europe, China, and Russia, centuries before the adoption of the Hindu-Arabic numeral system. The exact origin of the abacus has not yet emerged. It consists of rows of movable beads, or similar objects, strung on a wire. They represent digits. One of the two numbers is set up, and the beads are manipulated to perform an operation such as addition, or even a square or cubic root.

In their earliest designs, the rows of beads could be loose on a flat surface or sliding in grooves. Later the beads were made to slide on rods and built into a frame, allowing faster manipulation. Abacuses are still made, often as a bamboo frame with beads sliding on wires. In the ancient world, particularly before the introduction of positional notation, abacuses were a practical calculating tool. The abacus is still used to teach the fundamentals of mathematics to some children, for example, in Russia.
Designs such as the Japanese soroban have been used for practical calculations of up to multi-digit numbers. Any particular abacus design supports multiple methods to perform calculations, including addition, subtraction, multiplication, division, and square and cube roots. Some of these methods work with non-natural numbers (numbers such as 1.5 and 3⁄4).

John Napier devised Napier’s Bones, a manually
operated calculating apparatus. For calculating, this instrumentused 9 separate ivory strips (bones) marked with numerals to multiply and divide. It was also the first machineto calculate
using the decimal point system. The complete device usually includes a base board with a rim; the user places Napier's rods inside the rim to conduct multiplication or division. The board's left edge is divided into nine squares, holding the numbers 1 to 9. In Napier's original design, the rods are made of metal, wood or ivory and have a square cross-section. Each rod is engraved with a multiplication table on each of the four faces. In some later designs, the rods are flat and have two tables or only one engraved on them, and made of plastic or heavy cardboard. A set of such bones might be enclosed in a carrying case.

A rod's face is marked with nine squares. Each square except the top is divided into two halves by a diagonal line from the bottom left corner to the top right. The squares contain a simple multiplication table. The first holds a single digit, which Napier called the 'single'. The others hold the multiples of the single, namely twice the single, three times the single and so on up to the ninth square containing nine times the number in the top square. Single-digit numbers are written in the bottom right triangle leaving the other triangle blank, while double-digit numbers are written with a digit on either side of the diagonal.If the tables are held on single-sided rods, 40 rods are needed in order to multiply 4-digit numbers – since numbers may have repeated digits, four copies of the multiplication table for each of the digits 0 to 9 are needed. If square rods are used, the 40 multiplication tables can be inscribed on 10 rods. Napier gave details of a scheme for arranging the tables so that no rod has two copies of the same table, enabling every possible four-digit number to be represented by 4 of the 10 rods. A set of 20 rods, consisting of two identical copies of Napier's 10 rods, allows calculation with numbers of up to eight digits, and a set of 30 rods can be used for 12-digit numbers.

Pascal's calculator (also known as the arithmetic machine or Pascaline) is a mechanical calculator invented by Blaise Pascal in 1642. Pascal was led to develop a calculator by the laborious arithmetical calculations required by his father's work as the supervisor of taxes in Rouen. He designed the machine to add and subtract two numbers directly and to perform multiplication and division through repeated addition or subtraction.

Pascal's calculator was especially successful in the design of its carry mechanism, w hich adds 1 to 9 on one dial, and carries 1 to the next dial when the first dial changes from 9 to 0. His innovation made each digit independent of the state of the others, enabling multiple carries to rapidly cascade from one digit to another regardless of the machine's capacity. Pascal was also the first to shrink and adapt for his purpose a lantern gear, used in turret clocks and water wheels. This innovation allowed the device to resist the strength of any operator input with very little added friction.

Pascal designed the machine in 1642. After 50 prototypes, he presented the device to the public in 1645, dedicating it to Pierre Séguier, then chancellor of France. Pascal built around twenty more machines during the next decade, many of which improved on his original design. In 1649, King Louis XIV of France gave Pascal a royal privilege (similar to a patent), which provided the exclusive right to design and manufacture calculating machines in France. Nine Pascal calculators presently exist; most are on display in European museums.

The Complex Number Calculator (CNC) is completed	In 1939, Bell Telephone Laboratories completes this calculator, designed by scientist George Stibitz. In 1940, Stibitz demonstrated the CNC at an American Mathematical Society conference held at Dartmouth College. Stibitz stunned the group by performing calculations remotely on the CNC (located in New York City) using a Teletype terminal connected to New York over special telephone lines. This is likely the first example of remote access computing.
Konrad Zuse finishes the Z3 Computer	The Z3, an early computer built by German engineer Konrad Zuse working in complete isolation from developments elsewhere, uses 2,300 relays, performs floating point binary arithmetic, and has a 22-bit word length. The Z3 was used for aerodynamic calculations but was destroyed in a bombing raid on Berlin in late 1943. Zuse later supervised a reconstruction of the Z3 in the 1960s, which is currently on display at the Deutsches Museum in Munich.
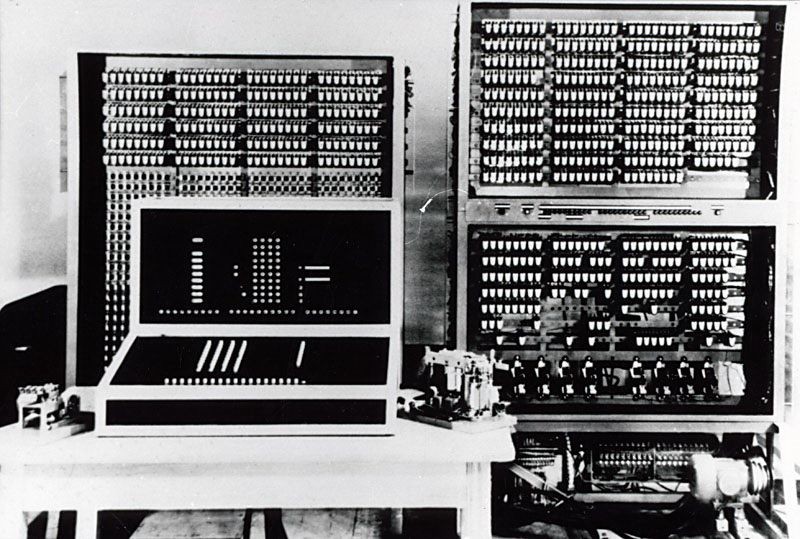The first Bombe is completed	Built as an electro-mechanical means of decrypting Nazi ENIGMA-based military communications during World War II, the British Bombe is conceived of by computer pioneer Alan Turing and Harold Keen of the British Tabulating Machine Company. Hundreds of allied bombes were built in order to determine the daily rotor start positions of Enigma cipher machines, which in turn allowed the Allies to decrypt German messages. The basic idea for bombes came from Polish code-breaker Marian Rejewski's 1938 "Bomba."